annadevelopment.blogg.se - Simplifying radicals program using ti connect

If we now expand each of these terms, we have Since (3x + z) is in parentheses, we can treat it as a single factor and expand (3x + z)(2x + y) in the same manner as A(2x + y). Now consider the product (3x + z)(2x + y). In the previous section you learned that the product A(2x + y) expands to A(2x) + A(y). Use the distributive property to multiply any two polynomials.In each of these examples we are using the distributive property. Notice each term is multiplied by 2x.Īgain, each term in the parentheses is multiplied by 3y 2Īgain, each term in the parentheses is multiplied by 3y 2.

Placing 2x directly in front of the parentheses means to multiply the expression in the parentheses by 2x. We now extend this idea to multiply a monomial by a polynomial. In the process of removing parentheses we have already noted that all terms in the parentheses are affected by the sign or number preceding the parentheses. If a polynomial has three terms it is called a trinomial. If a polynomial has two terms it is called a binomial. Special names are used for some polynomials. Generally, if there is more than one variable, a polynomial is written in alphabetical order.

Find the product of a monomial and binomial.Ī polynomial is the sum or difference of one or more monomials.

MONOMIALS MULTIPLIED BY POLYNOMIALS OBJECTIVES Remember, if an exponent is not written, an exponent of one is understood. Multiply 5 times 3 and add the exponents of x. To find the product of two monomials multiply the numerical coefficients and apply the first law of exponents to the literal factors.ĭo you remember the first law of exponents? Is not a monomial since the operation of addition is involved. Upon completing this section you should be able to:Ī monomial is an algebraic expression in which the literal numbers are related only by the operation of multiplication. If an expression contains the product of different bases, we apply the law to those bases that are alike. This fact is necessary to apply the laws of exponents. When we write x, the exponent is assumed: x = x1. This law applies only when this condition is met.Īn exponent of 1 is not usually written. Note in the above law that the base is the same in both factors. To multiply factors having the same base add the exponents.įor any rule, law, or formula we must always be very careful to meet the conditions required before attempting to apply it. These laws are derived directly from the definitions.įirst Law of Exponents If a and b are positive integers and x is a real number, then Now that we have reviewed these definitions we wish to establish the very important laws of exponents. Upon completing this section you should be able to correctly apply the first law of exponents. MULTIPLICATION LAW OF EXPONENTS OBJECTIVES We just do not bother to write an exponent of 1. It is also understood that a written numeral such as 3 has an exponent of 1. This can be very important in many operations. When we write a literal number such as x, it will be understood that the coefficient is one and the exponent is one.

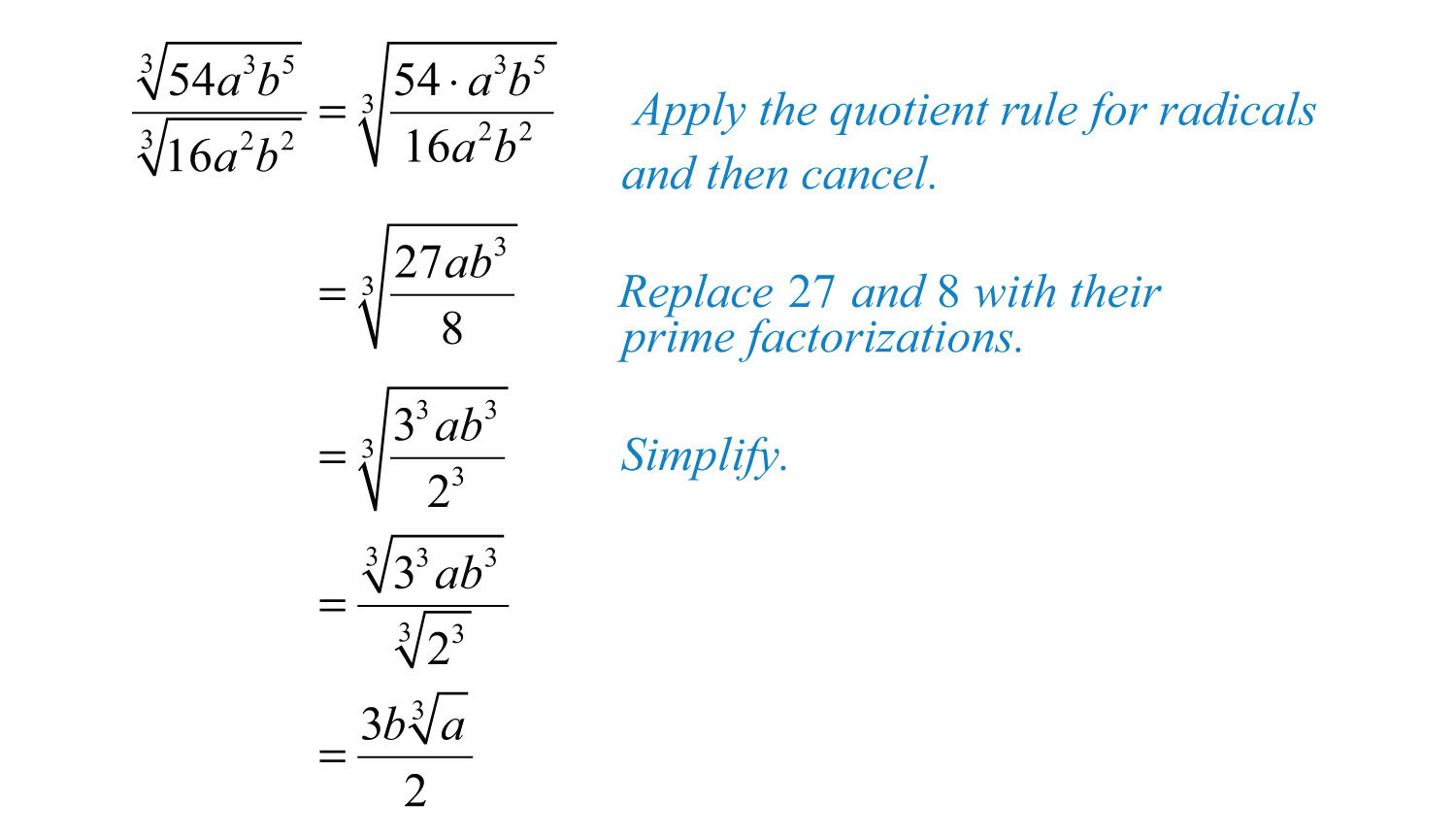
Many students make the error of multiplying the base by the exponent.For example, they will say 3 4 = 12 instead of the correct answer,

Note that only the base is affected by the exponent. Unless parentheses are used, the exponent only affects the factor directly preceding it. From using parentheses as grouping symbols we see thatĢx 3 means 2(x)(x)(x), whereas (2x) 3 means (2x)(2x)(2x) or 8x 3. Note the difference between 2x 3 and (2x) 3. An exponent is usually written as a smaller (in size) numeral slightly above and to the right of the factor affected by the exponent.Īn exponent is sometimes referred to as a "power." For example, 5 3 could be referred to as "five to the third power." Make sure you understand the definitions.Īn exponent is a numeral used to indicate how many times a factor is to be used in a product. When naming terms or factors, it is necessary to regard the entire expression.įrom now on through all algebra you will be using the words term and factor. Rules that apply to terms will not, in general, apply to factors. It is very important to be able to distinguish between terms and factors. When an algebraic expression is composed of parts to be multiplied, these parts are called the factors of the expression. In 2x + 5y - 3 the terms are 2x, 5y, and -3. When an algebraic expression is composed of parts connected by + or - signs, these parts, along with their signs, are called the terms of the expression.

Since these definitions take on new importance in this chapter, we will repeat them. In section 3 of chapter 1 there are several very important definitions, which we have used many times.